Moebius Basics

by Tuğrul Yazar | January 15, 2012 18:49

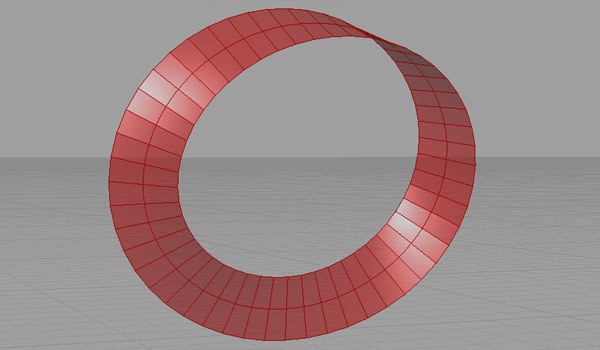
One of the most popular shapes in topology studies is the one-edge, one-face Moebius strip. Here is a basic definition that generates Moebius-like lofted surfaces. I say Moebius-like because, in Grasshopper, Rhino, or any NURBS surface method, I couldn’t manage to model this shape in its real topological singularity. The tricky part of this Grasshopper definition lies at the end, as I take the first segment of the surface, flip it, and attach it to the end of the segment list. This creates an illusion that the loft operation seems to create a one-edge surface.
There are very interesting sculptures created by using the Moebius strip. I plan to make such compositions (a component-based approach for example) as parametric models. Here is the Grasshopper definition you can download [2012_01_17-mobius][1].

[2]

Source URL: https://www.designcoding.net/moebius-frenzy/

---