Icosidodecahedron
Icosidodecahedron is an Archimedian Solid, a thing in between the Platonic Solids of Icosahedron (d20) and Dodecahedron (d12). It is a rectified version of an Icosahedron, constructed by dividing every edge into two equal segments and joining these segments to create a composition of equilateral pentagons and triangles. Archimedian Solids consist of at least two equilateral polygons, whereas Platonic Solids are constructed by only one. We’ll deduce an Icosidodecahedron from Icosahedron below;
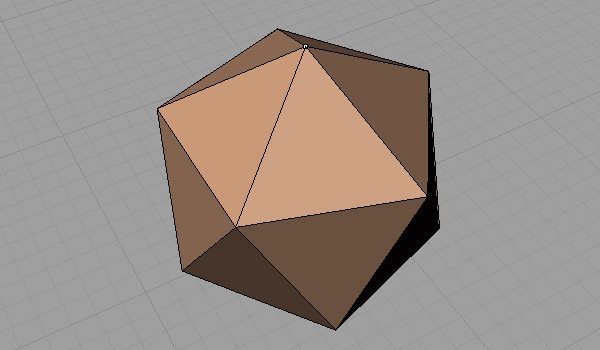
First, you should create an Icosahedron, the Platonic father of Icosidodecaheron. After that, all faces should be joined together to form a polysurface.
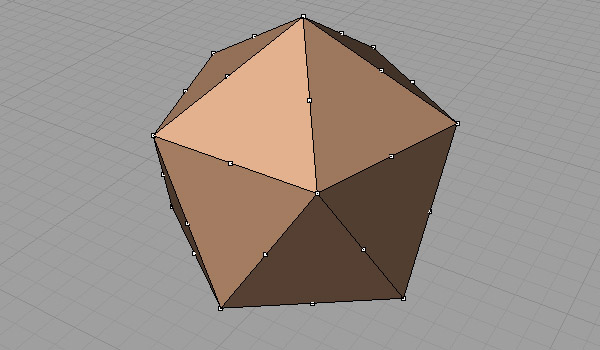
Then, extract all of its edges, using (Curve/Curve from Objects/Duplicate Edge) and divide them into two equal segments, using the (Curve/Point Object/Divide Curve by/Number of Segments/) command.
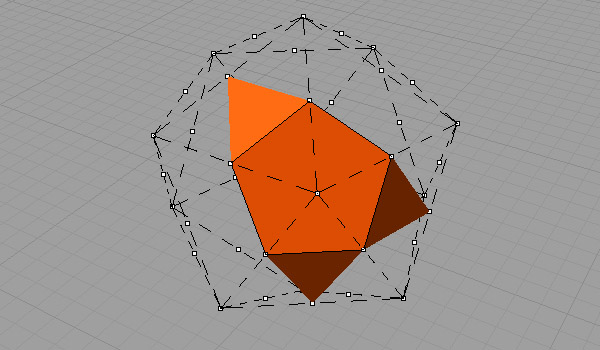
Now, the points should construct the faces of the Archimedian Solid.
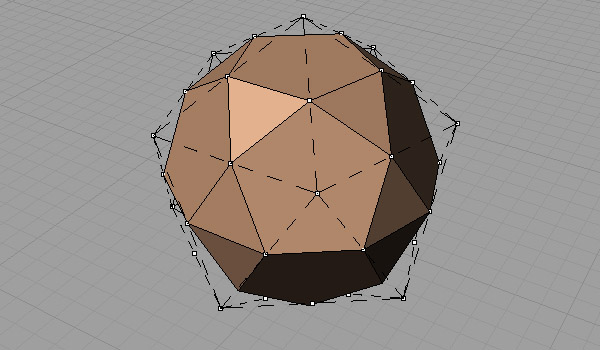
Here is the result:
Cite this post
Related Posts
Modeling Truncated Icosidodecahedron
December 15, 2024
In geometry, a truncated icosidodecahedron, rhombitruncated icosidodecahedron, great rhombicosidodecahedron, omnitruncated dodecahedron, or omnitruncated icosahedron is an Archimedean solid. It is one of thirteen convex, isogonal, non-prismatic solids constructed by two or more types of regular polygon faces. In this short tutorial, I am constructing an irregular truncated icosidodecahedron. It is not the regular Archimedean solid, but a rough approximation of it. I made this model to exercise the exploration of new polyhedra by using rectification and truncation operations. Nevertheless, here…
Modeling and Unrolling Icosidodecahedron
December 14, 2024
An icosidodecahedron is a polyhedron that is part of the family of Archimedean solids. It has 32 faces, consisting of 12 regular pentagonal faces of a dodecahedron and 20 regular triangular faces of an icosahedron. It is highly symmetrical, with the same arrangement of faces around each vertex. In this short tutorial video, I am modeling and unrolling an icosidodecahedron. This polyhedron is very interesting and is mostly used as a basis for more sophisticated designs. Its dual polyhedron is a rhombic triacontahedron,…
Modeling a Rhombicosidodecahedron
July 17, 2024
Modeling a rhombicosidodecahedron requires exploding and extending the faces of a dodecahedron and an icosahedron of the same edge length. We begin with both polyhedra centered at the same point. Then, we explode the faces of the dodecahedron and icosahedron outward from the center. We extend their planes while maintaining their orientation and shape. As these faces extend, they intersect and form new polygonal regions. Triangular and pentagonal faces emerge naturally from the extended faces of the dodecahedron and icosahedron,…
Modeling Stellated Icosahedron
December 14, 2024
Stellation of polyhedra refers to the process of extending the faces (or edges) of a polyhedron outward to form new, more complex shapes. These new shapes, called stellations, are created by adding extra vertices, edges, and faces that “project” outward from the original polyhedron’s structure. You can create an infinite number of new polyhedral forms, depending on how you extend the faces and edges. In this short tutorial video, I am modeling a stellated icosahedron. I use basic modeling commands…
Modeling Dual of Dodecahedron
December 14, 2024
A dual polyhedron is a concept in geometry where two polyhedra are related in such a way that the vertices of one polyhedron correspond to the faces of the other, and the faces of the first polyhedron correspond to the vertices of the second. The process of creating a dual polyhedron is called duality, and it applies to many regular, semi-regular, and some irregular polyhedra. In the case of Platonic solids, the cube and octahedron are duals of each other….