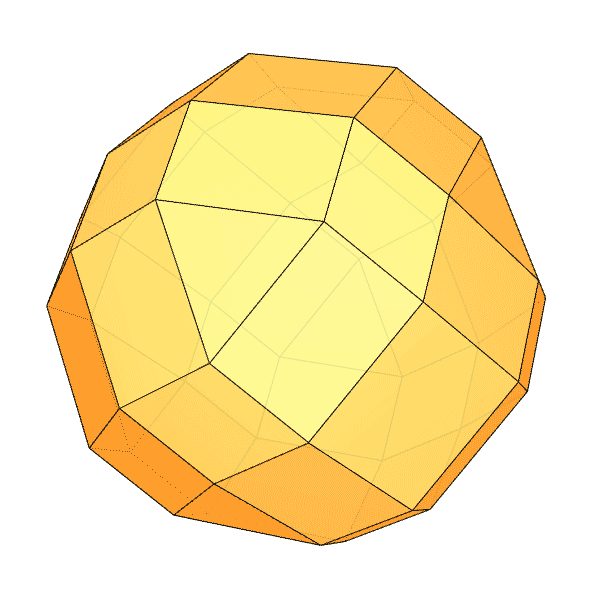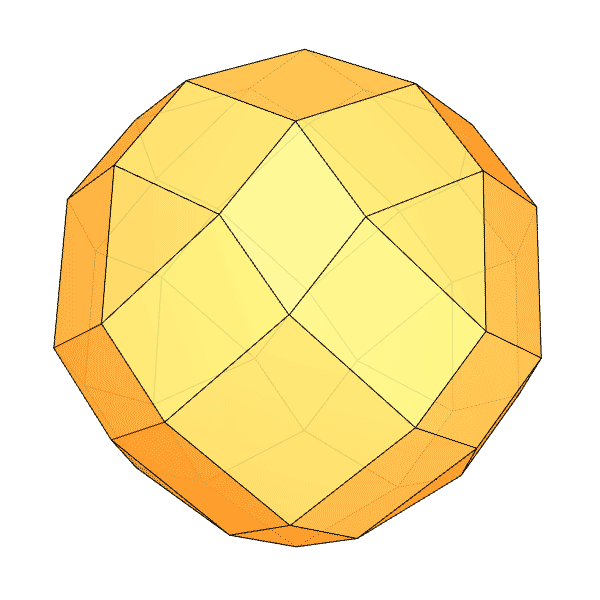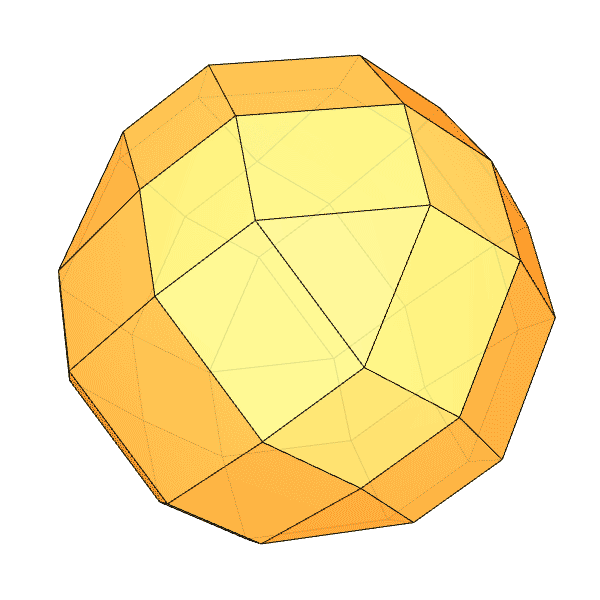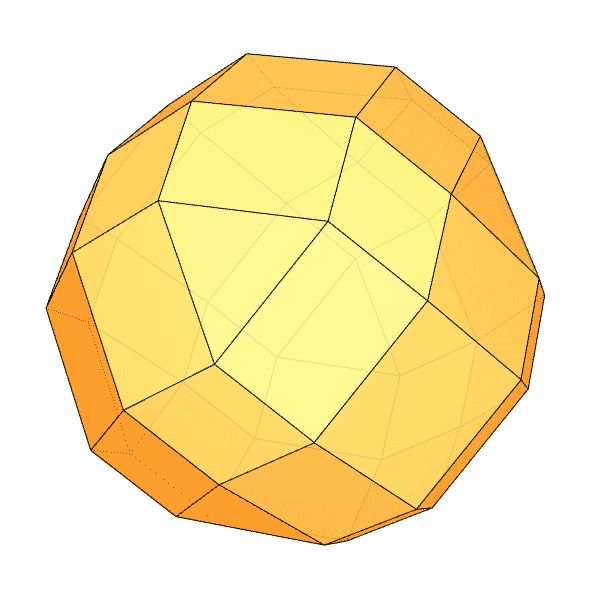
Chamfered Polyhedra
I had been researching Goldberg Polyhedra for a while. While exploring how to perform chamfer operations in Grasshopper, I found some interesting results. I want to share them with you. In this Grasshopper project, I reused my previous work, where I built a dodecahedron using the golden ratio. Essentially, all I did was add the Fillet Edge component. I don’t use this component much, so I learned it through this process. At one point, I realized I had created a Small Rhombicosidodecahedron. You can see my work on modeling the Archimedean solid in Rhino here. As I played with the parameters, I observed that many chamfered polyhedra emerged whose names I didn’t know. For example, when Factor=6.1892 and Radii=3.0, we get the shape you see at the bottom of this page. I named it “Augmented Rhombic Dodecahedron.” It has 12 rhombii, 12 squares, 8 triangles, and 24 rectangles.

Like every polyhedron with rhombii, I found chamfered polyhedra quite appealing. I don’t know why these polyhedra are so attractive to me. Every time I think, “I’ve seen them all, they’re starting to get boring,” new ones appear. Nowadays, we are under a bombardment of high-curvature surface aesthetics. Polyhedra, on the other hand, are relatively simpler and more modest. They represent an older form of geometry. For me, these are transition spaces related to the design of mathematics. Beautiful symmetry games are completed in a spherical form that guarantees completeness and wholeness. They are inward-looking but beautiful.
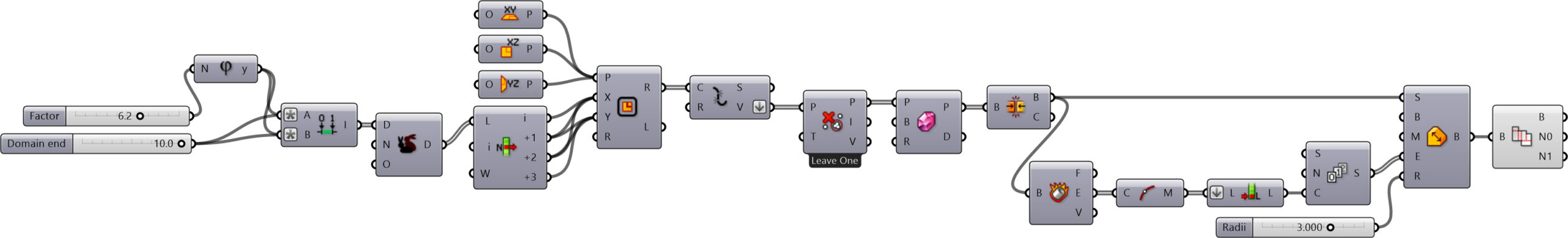
You can rebuild the Chamfered Polyhedra definition by looking at the diagram above. However, if you want to support my website by downloading my working Grasshopper file, would you like to be one of my Patreons? The link to my Patreon page includes the Grasshopper file for this project and more.