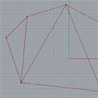
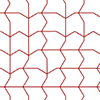
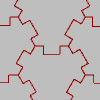
Instead of searching for an iterated and rule-based variety, this method captures instances of spatial deformation by transforming the hyperframe. This liberates us from a classical understanding of pattern deformations that are enframed within regular polygons, mostly rectangles or hexagons. Grasshopper has a built-in component to study such variety. The spatial Deform component gets vectors as inputs and transforms any given geometric object according to it. This website has also […]

Again, I continue with some simple solutions for Grasshopper. The surface split component gives all possible surfaces sliced with given curves. And it creates “invalid” curves with at least one open edge. I used this to perceive the closed regions within a given complex curve set. Just put the “Clean” component to erase the outer invalid surfaces and there remain the closed ones. However this time the question was where […]

This is a simple trick that shows the utilization of the “surface split” component in Grasshopper. It is used for detecting the inner regions of any given two-dimensional linework. Thus, it resembles the hatch boundary detection of AutoCAD and similar software. There is no built-in hatch component in Grasshopper. But maybe you can use this as a starting point if you want to develop it. The definition starts with drawing […]

Today’s tip is about two-dimensional curve-point calculations. It is very handy to use “closest point” components in Grasshopper. You can calculate distances and directions between curves, surfaces, and points. Then, place point objects in relation to the proximity of another object. However, there is no “farthest point” implemented yet. I tried to calculate the farthest point from a curve. First, I tried to translate the curve in a fashion that […]

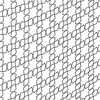
It has been a while since I didn’t post any patterns. Here is a beautiful one from the iconic design studio of William Huff. Crossover Parquet Deformation is a single-axis, line-based deformation algorithm, constructed on a regular quadrangular hyperframe, designed by Richard Lane at the Basic Design studio of William Huff in 1963. It presents two different parquet deformation sequences linked together. Thus, the designer created a transition between the […]

After becoming a ready-made component in Grasshopper, the Delaunay triangulation lost its popularity quickly. It used to be a nice problem of computational geometry for designers obsessed with scripting.  Last month, Benay reminded me of the method of circle checking. She showed her Rhinoscript that creates circles from point triplets and checks if a point is inside or not. Today I studied this in Grasshopper to see if I can […]

Kündekâri is an old woodworking technique, composed of interlocking parts without any glue or nail. It is primarily used in wooden doors and minbers inside Mosques. The interlocking system makes the whole structure very durable. I tried to model this technique using the Voronoi pattern, naming it Voronoi Kündekâri. Below you see a typical example of Kündekâri pieces and the resulting pattern. The interesting part of the Grasshopper model is […]

This is based on my failure of creating an optimum solution for planar polygonal subdivisions. There is a method called Tangent Plane Intersection (TPI), explained briefly here (sometimes similar algorithms are called “planar remeshing” and  “variational shape approximation”) which is effectively used in the Trada pavilion (here). I tried to implement a similar method using only native Grasshopper components and no recursion, but it quickly became much more complicated than […]

Below is a classical Truchet Patterns example we used to discuss in design computing class. Now, we can create a much faster and cleaner definition using the newly added Array component. The new version of Grasshopper brings several important features: Patch surfaces and date/time components. We used to implement a VB component to create simple clocks that measure temporal operations in Grasshopper. Parsing and executing MusicXML and related applications were […]

The story of Atatürk Cultural Center (in Turkish: Atatürk Kültür Merkezi – AKM) dates back to the 1930s. Auguste Perret designed the first version of the project and started the construction in the late ’30s. However, war and financial problems halted the construction. The project continued in the early 1950s with major updates and extensions. Faruk Akçer and Rüknettin Güney were responsible for this second version. Hayati Tabanlıoğlu designed the […]

The Parquet Deformation exercise is generally originated with William Huff. Huff conducted it at several schools of architecture since the 1960s. Huff defines the exercise as rooted in two analytical disciplines; monohedral tilings in geometry, and the continuous deformations in biological morphology. This is generally exemplified by D’Arcy Thompson’s and Albrecht Dürer’s studies. One of the student’s works of Huff, Trifoliolate is a single-axis, single-prototile hexagonal parquet deformation. It was […]

I’ve seen beautiful examples of similar compositions made using vector field components in Grasshopper. I just tried to make my own animate field lines to see how they float over force dynamics. In essence, these compositions could also be done using regular vector components but the field components make life much easier by merging different forces together rather quickly. Here is my Grasshopper definition (be careful it may slow the […]

This elegant and straightforward tiling geometry is credited to Dominican priest Sebastien Truchet in 1704 and was documented in a book titled “Memoir sur les Combinasions” (A Memoir on Combinations). After delving into the renowned Truchet Patterns in 2013, I revisited their three-dimensional tiling counterparts today. This served as a valuable exercise in geometry during my previous Design Geometry course. I believe it enhances one’s proficiency in mastering the technical […]

For the last 10 days, I’ve been searching for a proper algorithm for representing surfaces using planar shapes. It is obvious that triangulation is an answer but there is an interesting research topic of planar remeshing using shapes other than quads, hexagons, or any other regular polygons. Especially in computer graphics, such things refer to the optimization of models to decrease the load of GPUs. In the Grasshopper community, this […]

Is it possible to model a two-way parquet deformation using only native components of Grasshopper? In this definition, I limited myself to 10 of them. Parquet deformations are a very interesting and pedagogical topic to teach some of the basics of contemporary parametric modeling. This post explains a minimal parquet deformations algorithm in Grasshopper. However, this has its own limitations. You will see that the definition generates the attractor graph […]

Previous studies on trigonometric surface equations showed me an interesting alternative. This is the modification of the breaststroke surface definition. This time, I’m trying to fix the equation and change input values in a fashion that the waves of the surface are not symmetrical. Here are a few experiments on it; (Size, 44.8, X=10, Y=5, animating X’s from 0 to 32) (Size: 42, X=7, Y=5, animating X’s from 0 to […]

In Turkish, there is a strange word “baklava” that has many uses. According to Wikipedia: Baklava is a rich, sweet pastry made of layers of filo pastry filled with chopped nuts and sweetened with syrup or honey. It is characteristic of the cuisines of the former Ottoman Empire and those of Central and Southwest Asia. However, we should add that 150 gr. of baklava is 413 calories. Here is it […]

It all started with my new passion for origami tessellations, not much of origami, but the tessellation part. I was too lazy to fold it physically, nor model them using an engine such as Kangaroo. That would also be very unnecessary (and yes, very boring) to simulate a folding effort on the computer unless we lose our connection with the real world. Instead, I tried to look at a much […]

You might recall this type of parametric brickwork from architectural classics, such as the Programmed Wall by ETH Zurich and Gramazio Kohler Research, or the facade of the Mulberry House by SHoP Architects. Initially, I explored the simplest method for placing boxes on a surface, but this approach didn’t yield the correct layout. To improve it, I introduced gaps, which not only liberated the wall’s design but also opened up […]

Here are funny icons from Martin Berube (other icon sets of him here) if you plan to build your components or clusters in Grasshopper. It somehow became a fashion of Rhinoceros, to give names of animals to products, that at first seemed to be only the species in danger of extinction. (maybe I am wrong but it is a fact that %85 of Black Rhinos were killed in the past […]